HOME | DD
batjorge β Building Boxes
by-nc-sa
#boxes #mb3 #4dobject #3dfractal #digital #fractal #mathematical #3dartwork #mandelbulb3d
Published: 2016-02-27 18:41:51 +0000 UTC; Views: 2872; Favourites: 97; Downloads: 0
Redirect to original
Description
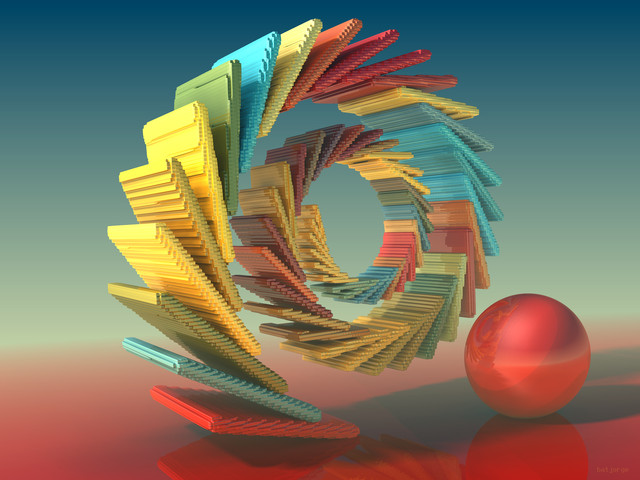
Mandelbulb 3D 189._PolyFold-sym, _sqr_4D, Makin4D p2b, QuatPow and HeightMapIFS in DEcombinate.
Related content
Comments: 70
Thank you! Good old experimental ones
π: 0 β©: 0
Out of curiosity, is it possible to isolate one of the boxes from the others, or does the nature of the fractal mean you have to see them in sextuplets?
π: 0 β©: 1
Yes you can easily separate them by turning off _PolyFold-sym or even to create more 
π: 0 β©: 1
Do you think I could see one by itself?
π: 0 β©: 1
Took several hours to render it, don't think will do it again 
π: 0 β©: 1
Admittedly, I was planning on using it for a Magic card, though the original deviation will also fill that role.
π: 0 β©: 1
Hope that never will happen. My works are not allowed for commercial purposes. Read CCL below. Had some serious issues with people using them for magic Cards. Took time to take it down, but wouldn't mind repeat the process.
π: 0 β©: 1
Oh, you don't have to worry. This is a solely non-commercial endeavor. These Magic cards are in now way affiliated with Wizards of the Coast. ^-^' I apologize for the confusion.
π: 0 β©: 1
Glad you approve Luca
π: 0 β©: 0
Very kind
π: 0 β©: 0
π: 0 β©: 1
Did just one single lonely box and thought I could multiply them 
Thank you a lot Sabine
π: 0 β©: 1
Awww how very nice for the box, you've made some friends for it! 
Of course I will now have to try and do something like it 
Always a great pleasure, Jorge!
π: 0 β©: 0
Alea iacta est - Excellent graphic, JorgeΒ Β !!
π: 0 β©: 1
You're welcome, JorgeΒ Β !!
π: 0 β©: 0
I want to play with them!
Well done, Jorge!
π: 0 β©: 1
π: 0 β©: 1
Keep up the fantastic work!
You do inspire me with M3D! Β
You are most welcome!
π: 0 β©: 0
Fantastically beautiful work! Reminds me on a wonderful, magical craps !
π: 0 β©: 1
Oh wow, Jorge--this is really, really great!Β Β Β Β Β Β
π: 0 β©: 1
You're very welcome, Jorge!Β
π: 0 β©: 0
Very well deserved and you're welcome!
π: 0 β©: 0
Cool Awesome! Cube/boxes here and such Pretty colors also.
π: 0 β©: 1
Glad you like them Barbara 
π: 0 β©: 1
They are sure different from any I have seen also A keeper this one is.
and Most welcome.
π: 0 β©: 0
Excellent! As usual, you are very inventive and unpredictable... Β
π: 0 β©: 1
I appreciate very much your comment
π: 0 β©: 1
| Next =>