HOME | DD
 DinkydauSet — Spiral iteration Julia ghost
by-nc-nd
DinkydauSet — Spiral iteration Julia ghost
by-nc-nd
#julia #mandelbrot #morphing #set
Published: 2019-08-18 23:26:13 +0000 UTC; Views: 862; Favourites: 39; Downloads: 12
Redirect to original
Description
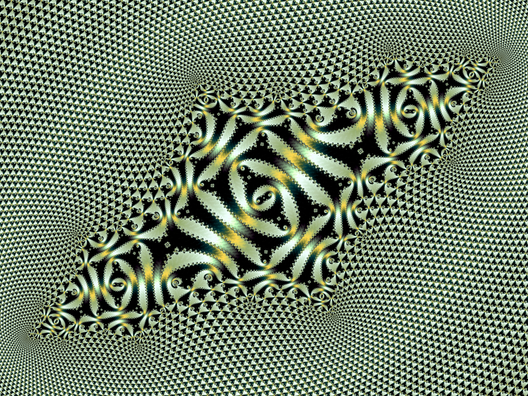
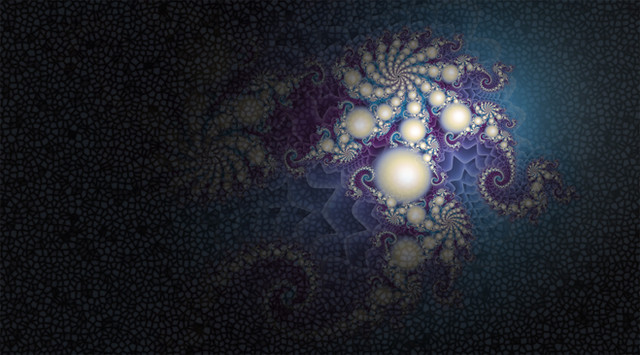
Mandelbrot setrendered with Mandel Machine
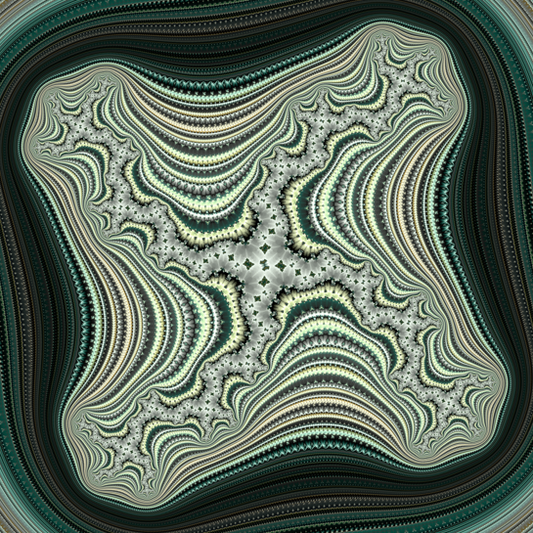
Arms of spirals can be thought of as infinitely long rows. The repeating parts look differently for each spiral, and some spirals have more arms than others, but they're all infinitely long rows of something. Idea: morph the spiral by zooming in on the first "thing" of an arm, then on the second "thing", then the third and so on. The only problem is that after a morphing, the number of spirals becomes doubled, so a choice has to be made: in which of the spirals to continue? The first two times the choice doesn't matter. At first there is only one spiral so only one can be chosen. After that any choice of the 2 spirals has the same effect. It's only at the third and later morphings that the choice matters. For this image I chose to skip one spiral, as counted from the center.
Skipping one spiral each time in practice appears to be a case of repeatedly choosing almost (but not) exactly the same location (compared to the center of the figure) to zoom in on, which (if it was exact) gives the shape of a Julia set as the number of morphings goes to infinity. In the terms I used in the journal Simulating Julia morphings it is (almost exactly) the "same" Julia morphing. So that's why it looks like a Julia set, even though it isn't one. It's a finite approximation using finitely many Julia morphings (and again: not exactly the same one each time, but close enough that the result does look like a Julia set). If you look closely, you can see that the boundary of the Julia set blends with the surroundings. That's why I call it a ghost. It's similar to what happens in the image Fully connected partitioning .
For the same reason, Evolution of trees and images like Triangle tiling with layered julia morphing look like Julia sets, and also this image can be thought of as an evolution.
A hint about what's coming next: The only property of spirals that's being used here is that they're sequences of things. That means the same can be done with many other shapes that are like sequences, such as rings around minibrots. They're highly rotationally symmetrical, for example 1024-fold. That's like a 1024 long sequence of the same things. There is a lot that can be done with this idea, but just using various kinds of spirals and rings could get boring so I will make at least a few more images with this but maybe not more than 2 or 3.
Magnification:
2^2674.558
1.3249001310955370696105910200074 E805
Coordinates:
Re = -0.7647372261520127639231804540823722731471203247213111158136673051498145032842233786198794647295610985947573674159150154664178496866904409820470457511246230330918278978921469988249221548887223453010599113257862108727216274897185345886145198943045105657143359999852064697072692559483866593547976111494260389522287339010226203992277572164596425132432290051294665511223114666123569367036498014961537509444720767831551760984108884338670927156046859398349260131420733596425818441807386306492785338361116698395022092725874464467356643573664961158217950650835068879195570856875898940411600552331881027522800250021622661013477034391938598990160725958031893494072452572559765875117875159425666065389609893313576571300801230611352057523453267435714181018443442578020370776454030462332421783448202235465816764979306188707160
Im = 0.0850452773946503334782488144975115657759885466678646590651344852105209461054082887778637356715802850143376262846370214950193868450216729093274888251528923430957390792603863421422557972816252858186270003992948577804732122190781482718517023874517064248672801935989732622084793593428317898751461668586558047891858095450113574787225695749133897041520884633173939196770569715419417442735272237318851121253995204275813187798421407414800355314601525511699747599802537228602395514638136286853691271250562418092825000208717078619099821216813994191637201227254970582623937602085536667753202241379676804761118401010249970426788892529117031295532668603947342149955067254362663536384534915194016906648511083733749044417301139639541809030789110412531783734672107305783703272243636813571576588311365643573972287453678048933165
Related content
Comments: 9

Beautiful and stunning work! Once again, how incredibly beautiful!
👍: 0 ⏩: 1

👍: 0 ⏩: 0

👍: 0 ⏩: 0