HOME | DD
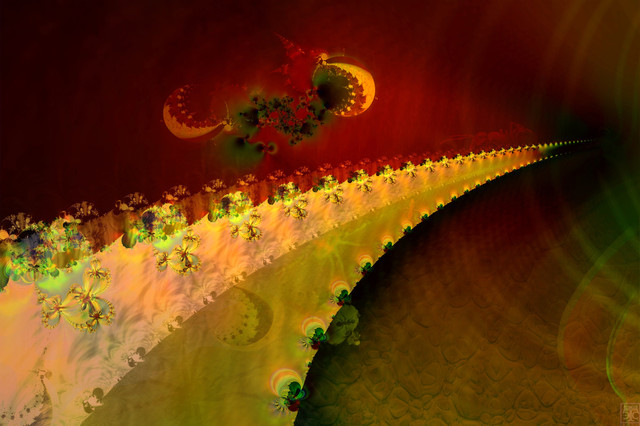
 FractalMonster — Bouncing in the Singularity1
by-nc-sa
FractalMonster — Bouncing in the Singularity1
by-nc-sa
Published: 2012-09-17 20:24:24 +0000 UTC; Views: 469; Favourites: 14; Downloads: 15
Redirect to original
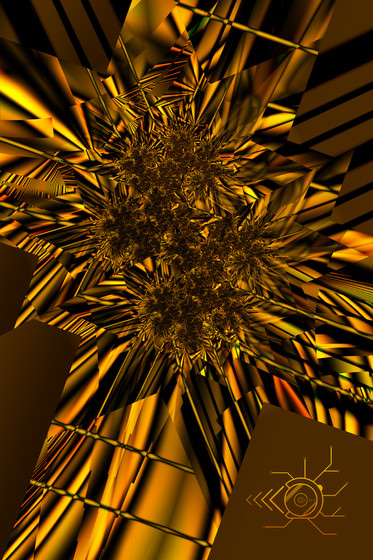
Description
Download for 1960x1470Note: This is only a 1-layer image. The displaying of more than 1 subset is due to the techique of SetBorders. See article 19b in my Chaotic series .
The 16 dimensional Nonic parameter space.
*a_real = #pixel (horizontal before being rotated 90 degrees counter clockwise)
*a_imag = 0
*b_real = 0
*b_imag = 0.7
*c_real = 0
*c_imag = -0.7
*d_real = 0
*d_imag = 0.7
*e_real = 0
*e_imag = -0.7
*f_real = 0.7
*f_imag = 0
*g_real = -0.7
*g_imag = 0
*h_real = 0
*h_imag = #pixel (vertical before being rotated 90 degrees counter clockwise )
The motive is zoomed in to a structure in the right side in the earlier motive Portal to the Promised Land
Software: Ultra Fractal.
Color routine: Continuous Potential Method (CPM).
Formula: Nonic Parameterspace3 in the sp3-module written by my dear friend Greenseng here at DeviantART. All his modules, as well as mine can be downloaded from here
Below the parameter file, play and have fun





BouncingInTheSingularity1 {
fractal:
title="Bouncing in the Singularity1" width=640 height=480 layers=1
credits="Ingvar Kullberg;9/17/2012;Stig Pettersson;3/13/2011"
layer:
caption="Layer 1" opacity=100 mergemode=difference method=multipass
mapping:
center=1.169208217505/-0.09825129463825 magn=512.96615 angle=270
formula:
maxiter=5000 filename="sp3.ufm" entry="NonicParameterspace3"
p_PlottedPlane="15.(a-real,h-imag)" p_M=NCL p_SetBorders=yes
p_hide=yes p_areal=0.0 p_aimag=0.0 p_breal=0 p_bimag=0.7 p_creal=0
p_cimag=-0.7 p_dreal=0 p_dimag=0.7 p_ereal=0 p_eimag=-0.7
p_freal=0.7 p_fimag=0.0 p_greal=-0.7 p_gimag=0.0 p_hreal=0.0
p_himag=0.0 p_xrot=0.0 p_yrot=0.0 p_xrott=0.0 p_yrott=0.0
p_xrotu=0.0 p_yrotu=0.0 p_xrotv=0.0 p_yrotv=0.0 p_xrotr=0.0
p_yrotr=0.0 p_xrots=0.0 p_yrots=0.0 p_xrota=0.0 p_yrota=0.0
p_xrotb=0.0 p_yrotb=0.0 p_xrotc=0.0 p_yrotc=0.0 p_xrotd=0.0
p_yrotd=0.0 p_xrote=0.0 p_yrote=0.0 p_xrotf=0.0 p_yrotf=0.0
p_xrotg=0.0 p_yrotg=0.0 p_xroth=0.0 p_yroth=0.0 p_zrot=0.0
p_LocalRot=no p_diff=no p_bailout=1000.0 p_dbailout=1E-6
inside:
transfer=none
outside:
transfer=sqrt filename="spr.ucl" entry="ContinousPotential"
p_auto=yes p_auton=2.0 p_n=1.0 p_numfact=1.0 p_scale=1.0 p_smooth=no
p_epsilon=0.5 p_illustr=no p_limiton=no p_limit=0.1 p_index3=0.0
p_index1=0.99 p_index2=0.0 p_speed=0.5 p_acc=1.0 p_clog=yes
p_power=9.0 p_reversed=no p_test=no p_testvalue=0.7 p_index4=0.29
gradient:
smooth=yes index=30 color=17807 index=42 color=62720 index=73
color=1912362 index=97 color=65530 index=148 color=329215 index=211
color=15335546 index=280 color=32
opacity:
smooth=no index=0 opacity=255
}
Related content
Comments: 15

i really like this one! i see many things in it, like an neverending road with trees on both sides during some kind of sunset
👍: 0 ⏩: 2

That's also my view 


👍: 0 ⏩: 1

not really,. but i can place it :3
👍: 0 ⏩: 1



First: Check out the zoom sequences (only images) from page 4 in the pdf's [link] and [link] . The end motives are my deviations [link] and [link]
Second: In my journal [link] you have some links to short cool fractal animations I stumbled over at YouTube
Third: If you wanna try some free simple fractal software, go to my journal [link] . You don't need to know the math behind
Fourth: Wikipedia has a nice site [link]
Fifth: Maybe you would appreciate my "Chaotic series of fractal articles" [link] if you have a little theoretical interest
Hope you don't feel spammed
👍: 0 ⏩: 1

Ofcourse not! I really dont minde some information at all.
i am going to look at it ^^
👍: 0 ⏩: 1


.. and from the middle of the nineties there is a fantastic TV program about fractals, Colors of Infinity by Arthur Clarke
👍: 0 ⏩: 1

yeah i am glad it remains. because i already forgot half of what i read.
👍: 0 ⏩: 1


👍: 0 ⏩: 0

or it is me, having too much imagination again.. xD
👍: 0 ⏩: 0

Absolutely brilliant!!! 


Fantastic coloring - very sensitive close to the set. And the total motive is fantastic.
👍: 0 ⏩: 1

I am also very satisfied with the coloring 

👍: 0 ⏩: 1

Sorry for the misspellings
👍: 0 ⏩: 0