HOME | DD
 FractalMonster — Conjuring the Hydra19
by-nc-sa
FractalMonster — Conjuring the Hydra19
by-nc-sa
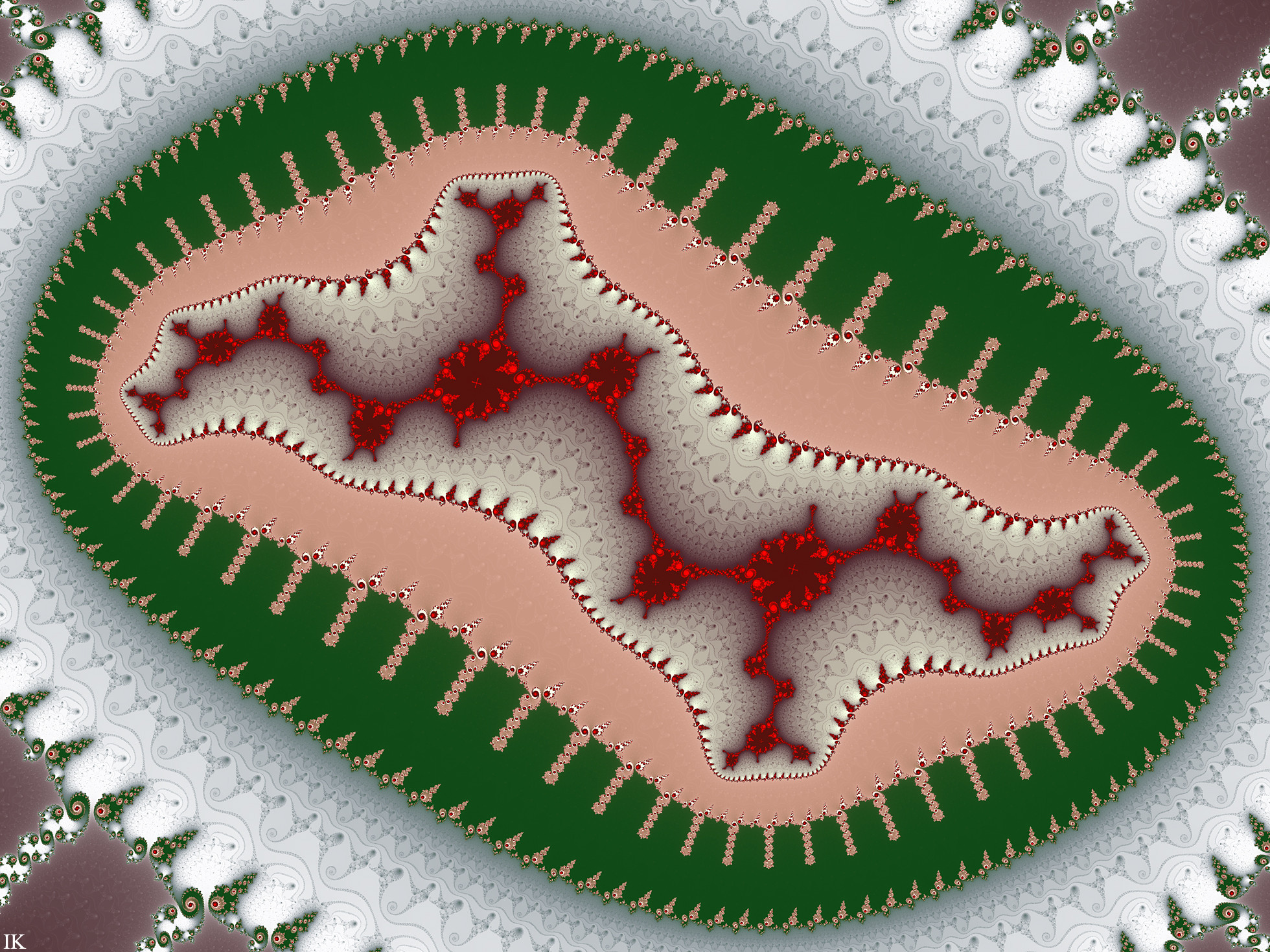
#mandelbrot #set
Published: 2020-01-22 19:46:52 +0000 UTC; Views: 325; Favourites: 23; Downloads: 3
Redirect to original
Description
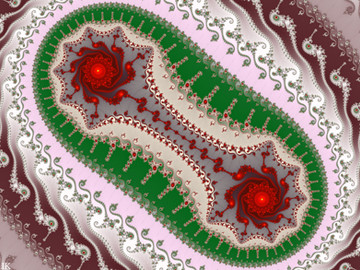
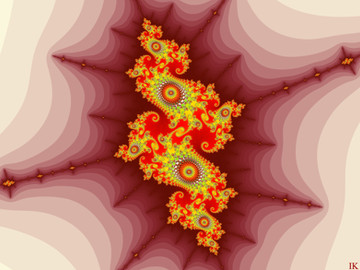
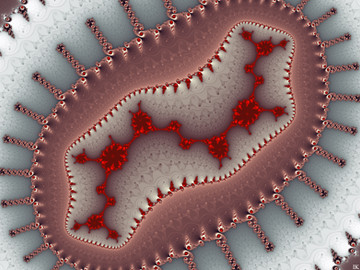
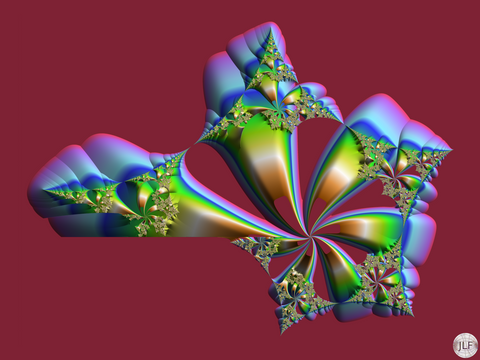
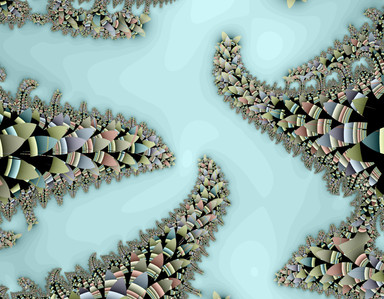
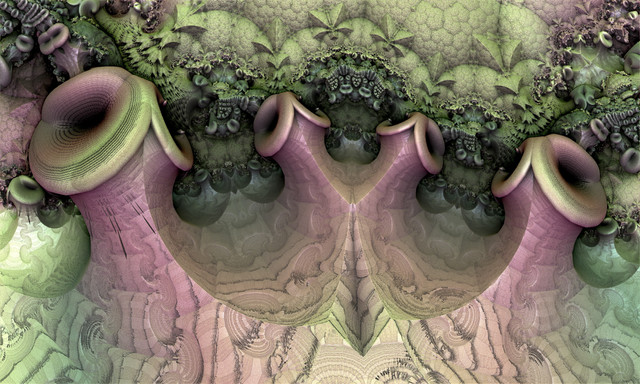
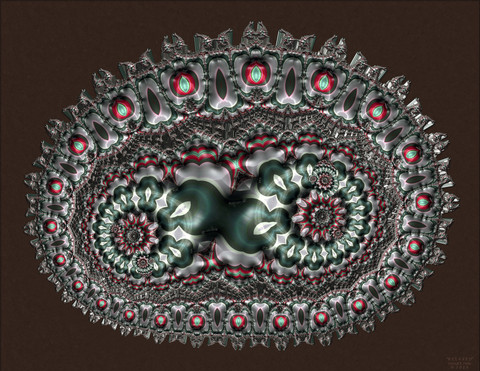
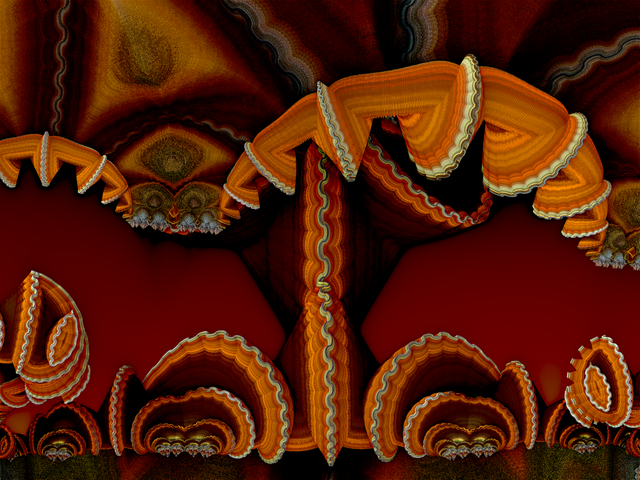
From the very last one in the series When the Eel Became a HydraMy recent uploads have been yet another series of what is called ”Julia morphing”. In order to know what is meant by that:First: Read the article 12) Julia-like barriers in my Chaotic series of fractal articles (if the link doesn’t open, open a new tab en paste klippan.seths.se/fractals/articles into the address bar). This article consists of more pretty illustrations than text and requires no mathematical knowledge







Second: Keep track of the final question: ”Not only in the centers of each barrier there are smaller systems of barriers, but in every gap where 2, 4, 8 etc spirals meet. Are these smaller systems to be found there in any way different? This question I leave to the diligent reader to explore.”
The answer of that question will give rise to what is called Julia morphing







Note: Also near copies of the Mandelbrot set , that is .
I've for a whole ago took the challenge to make further zoom-ins. This one i a
deep in-zoom into the middle left tip, a little bit down the center. The rendering
of the originally 6400x4800 image took about 45 hours using the setting "fast"
in the perturbation algorithm







Formula: Standard Mandelbrot set.
Software: Ultra Fractal 6.02.
Below the UF parameter file. Play and have fun 
ConjuringTheHydra19 {
fractal:
title="Conjuring the Hydra19" width=800 height=600 layers=1
credits="Ingvar Kullberg;1/9/2020"
layer:
caption="Layer 1" opacity=100 method=multipass
mapping:
center=0.25827112860511676746747306512794017578496060968477025703282\
51131782821944337387518577181242496316671551786060673084182244243799\
43547080926863373279352954932323730438353900794219195729917280201851\
02291262024253022966773338928478804755498563476553250761879210427888\
7715/-0.001215985529211351830663646819214939224843803858225410181643\
58109784802824769016663761833996805620074798224061671669030406818194\
92681445425340434566426043963043373257801914171757903517156792889248\
62477510529412868855177031151641811971273466515119996496305993337135\
11425 magn=4.6546546E254
formula:
maxiter=10000000 percheck=off perturbprecision=fast
filename="Standard.ufm" entry="Mandelbrot" p_start=0/0 p_power=2/0
p_bailout=100
inside:
transfer=none
outside:
density=0.1 transfer=sqrt filename="sp.ucl" entry="Basic2" p_up=0.0
p_down=0.0 p_lp=2.0 p_lk=1.0 p_noblurr=no p_p=1.0 p_k=0.01
p_hightrap=0.0 p_lowtrap=0.0 p_mask=no p_solid=yes p_colindex=0.0
gradient:
smooth=yes rotation=-132 index=6 color=9544661 index=17
color=14942198 index=21 color=3024720 index=38 color=527714 index=55
color=1014 index=111 color=254 index=-208 color=57087 index=-98
color=16777215 index=356 color=2498882 index=-26 color=16579579
index=376 color=9078905 index=-12 color=1460751
opacity:
smooth=no index=0 opacity=255
}
Related content
Comments: 11

Really glad you like this vry long rendering (45 hours) motive
.. and thanks for the 
👍: 0 ⏩: 0


.. and thanks for the 
👍: 0 ⏩: 0



BTW, heard about fractals before?
.. and thanks for the 
👍: 0 ⏩: 1

Yes I have and yours are very clean!
👍: 0 ⏩: 1



IF you wanna try some free simple fractal software of "Mandel an Julia" type, go to this journal. You don't need to know the math behind 
(if the links to my external pages don't work, check out this journal)
Maybe you would appreciate my Chaotic series of fractal articles (<- click) if you have a little theoretical interest 
Regarding deep zooms in the Mandelbrot set, if you check out my deviation Cauliflowerfort and click the link under "Artist's Comments", from page 4 you can follow the entire zoom sequence in 28 steps 
In this journal you have some links to cool fractal animations I stumbled over at YouTube. Also check out the zoom videos in this journal 
.. and from the middle of the nineties there is a fantastic TV program about fractals, Colors of Infinity by Arthur Clarke 

(the above info is recycled)
👍: 0 ⏩: 0



Worth 45 hours rendering time
👍: 0 ⏩: 1

My pleasure! Very worth the time!
👍: 0 ⏩: 0