HOME | DD
 FractalMonster — DemoCCL+SetBorders
by-nc-sa
FractalMonster — DemoCCL+SetBorders
by-nc-sa
Published: 2008-03-06 22:12:05 +0000 UTC; Views: 465; Favourites: 15; Downloads: 26
Redirect to original
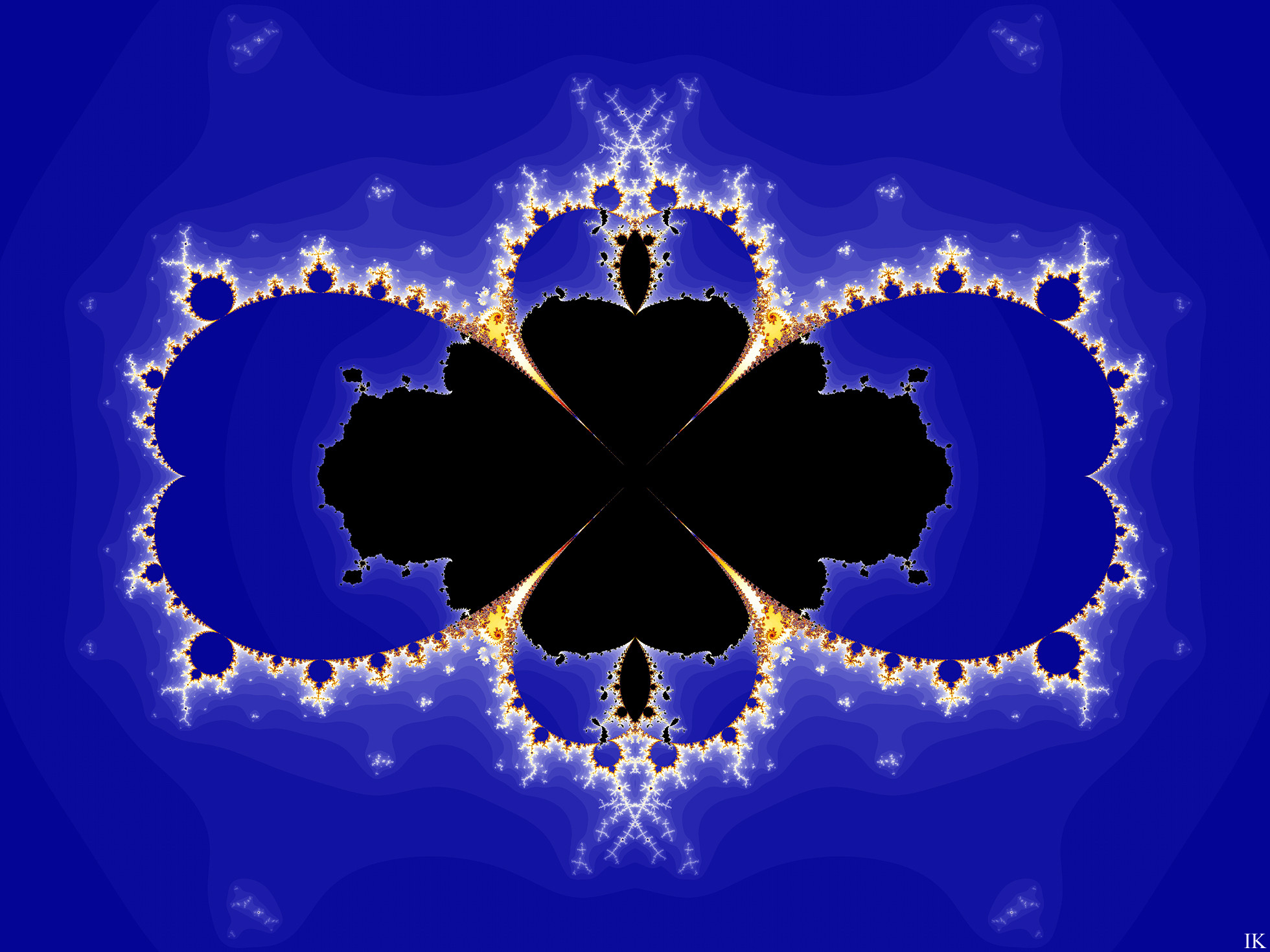
Description
Updated to 1960x1470 resolution when downloaded
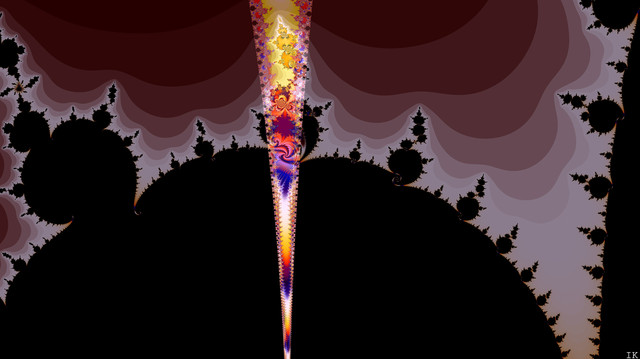
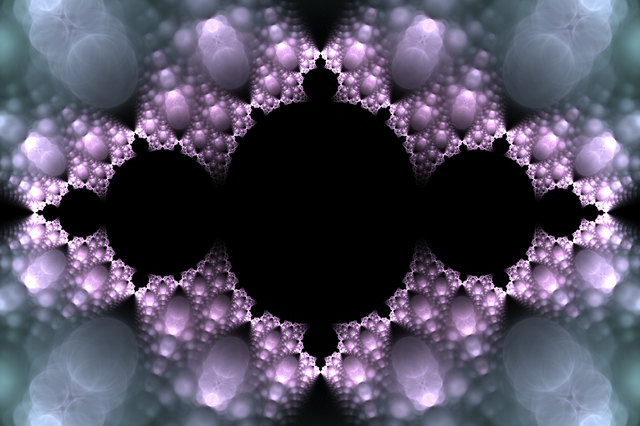
This is a comment to article 19b in my Chaotic series of fractal articles.
This motive is the same as Figure 9 in article 19b. Further comments see that article.
CCL means Cubic Connectedness Locus and is that set that is common to the sets M+ and M-. SetBorders is a facility that visualizes the contours of the parts of the sets that are not common. . Further comments see the above-mentioned article.
Question: Why is the position along the non-plotted real a-axis defined with a precision of 5 decimals (a_real = 0.57735)?
Answer: That’s necessary in order to obtain four ravines ending up in a single point (at b_real = 0 and b_imag = 0)
Question: But how in the heck have you found out that number (0.57735) that results in these ravines ending up in a single point?
Answer: Hehe well, I keep that as a little secret for a while 


Below the parameter file. Play and have fun
DemoCCL+SetBorders {
fractal:
title="DemoCCL+SetBorders" width=800 height=600 layers=1
credits="Ingvar Kullberg;6/14/2018"
layer:
caption="CCL+SetBorders" opacity=100 method=multipass
mapping:
center=0/0 magn=1.3
formula:
maxiter=10000 percheck=off filename="sp3.ufm"
entry="CubicParameterspace3" p_PlottedPlane="6.(b-real,b-imag)"
p_M=CCL p_SetBorders=yes p_hide=yes p_areal=0.57735 p_aimag=0.0
p_breal=0.0 p_bimag=0.0 p_xrot=0.0 p_yrot=0.0 p_xrott=0.0
p_yrott=0.0 p_zrot=0.0 p_LocalRot=no p_diff=no p_bailout=100.0
p_dbailout=1E-6
inside:
transfer=none
outside:
transfer=linear
gradient:
smooth=yes index=0 color=8716288 index=100 color=16121855 index=200
color=46591 index=300 color=156
opacity:
smooth=no index=0 opacity=255
}
Related content
Comments: 16

M a o förstummad
Man måste inte sätta or d på allt
👍: 0 ⏩: 1

I always have
I was surprised you didn't forced me to zoom into one of the 4 thin ravines, requiring a very hard computer calculation (which mostly is the case regarding interesting views). In fact this deviation was aimed for mere theory and ..
.. the 4 thin ravines is collapsing into a single point in the very middle, the only point where the 4 continents are connected
👍: 0 ⏩: 1

Nah, it's good as it is right now, there's no need for enlarging the image when the motive is already appealing.
👍: 0 ⏩: 1

Yeah
And in this case not much more will be discovered. We will see
👍: 0 ⏩: 0

That is way cool, finally someone who knows the math!!
👍: 0 ⏩: 1

Yeah, the very little bit of the very basic math I know is VERY fascinating 

👍: 0 ⏩: 0

Tack Johanna 
👍: 0 ⏩: 1

Woho! ännu mer blått XD
👍: 0 ⏩: 1

Haha, det blir väl till att göra särskilda johanna-versioner med bara nyanser av blått
👍: 0 ⏩: 1