HOME | DD
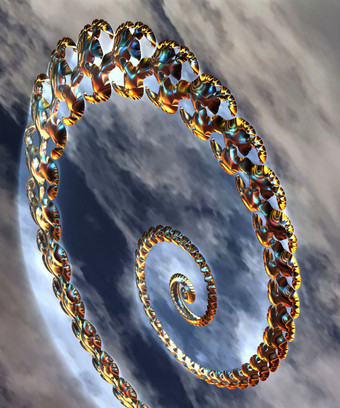
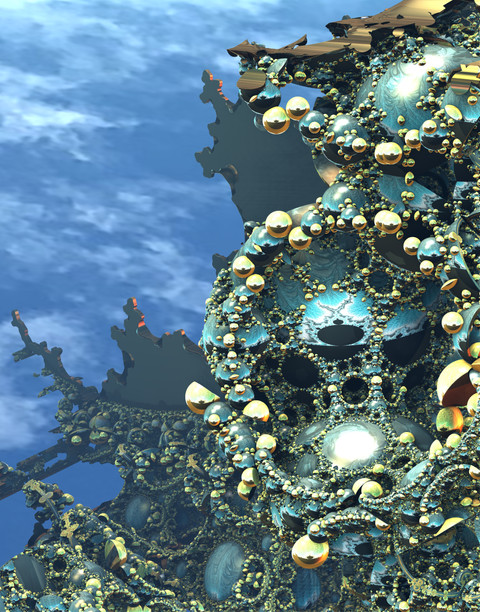
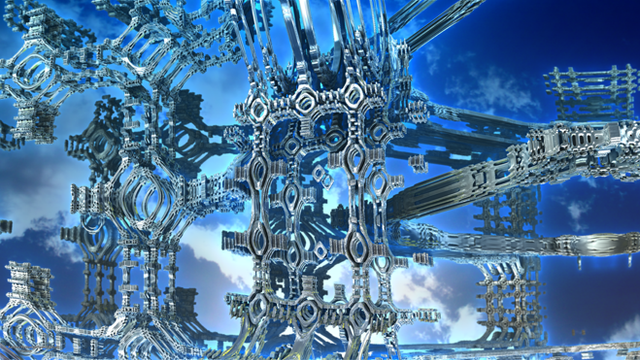
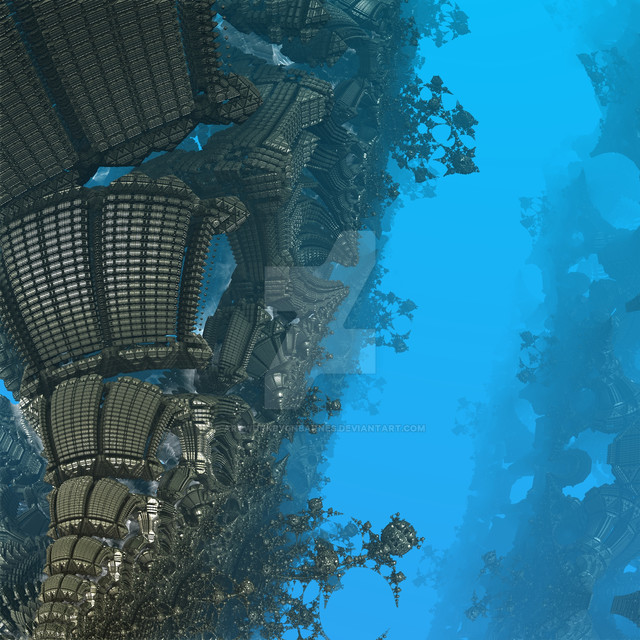
Metafractals — Tetrabrot Tower - Zoom in, No. 01
#digitalart #fractalart #fractals #hybrid #klein #mandelbrot #tetrabrot #3dfractals #mb3d #3dmandelbrot
Published: 2019-08-12 17:52:39 +0000 UTC; Views: 1075; Favourites: 55; Downloads: 0
Redirect to original
Description
Tweak of Tetrabrot – Kleinian Ed. by Metafractals . Hybridization by decombination between the Tetrabrot (Power 2) and a 3D Kleinian IFS. In multicomplex dynamics, the Tetrabrot is a 3D generalization of the Mandelbrot set. Discovered in 2000, this 3D Fractal shape can be interpreted as a 3D slice of the 8D tricomplex Mandelbrot set.
Tool: Mandelbulb3D (MB3D: KaliLinCombSSE2sm & JosKn-KleinIFS, Lightmap FraxPro)
Related content
Comments: 18
👍: 1 ⏩: 1
👍: 0 ⏩: 1
👍: 1 ⏩: 0
👍: 0 ⏩: 1
Thank you so much Margaret...!! ✨
👍: 0 ⏩: 0
Seems like coral reef has taken over some ancient ruins.
👍: 0 ⏩: 1
Thank you very much for this wonderful poetic interpretation.
👍: 0 ⏩: 0
Zooming in on this Tetrabrot Tower is fabulous! Superb Fractal Art!!
👍: 0 ⏩: 1
Thank you so much...!! 🌻
👍: 0 ⏩: 1
👍: 0 ⏩: 0
Cool! Thank you very much...!! ✨
👍: 0 ⏩: 1
This is like finding an ancient and alien monument at the top of a very high mountain peak. Superb art and in the final full view, it is magnificent!
👍: 0 ⏩: 1
Thank you so much Lea...!! ✨
👍: 0 ⏩: 1