HOME | DD
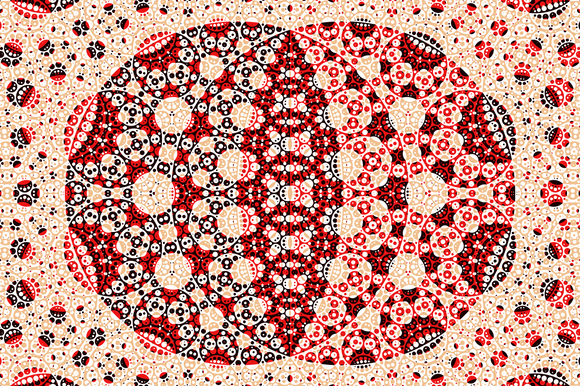
Vladimir-Bulatov — M.C.Escher Circle Limit III in a rectangle
by-nc-sa
Published: 2012-01-27 23:59:20 +0000 UTC; Views: 7768; Favourites: 29; Downloads: 749
Redirect to original
Description
Circle Limit III with fractal boundary and rectangular limitRelated content
Comments: 11
Would it be ok for me to use this for a while on my facebook page?
👍: 0 ⏩: 1
Yes, it would be OK.
Some form of credit would be appreciated
👍: 0 ⏩: 0
Your deviation has been featured in my journal this week. [link]
👍: 0 ⏩: 1
You are very welcome.
👍: 0 ⏩: 0
Wow. I wish I knew the math behind this, I have a hard enough time with the circular poincare disk! Is this hyperbolic or fractal?
👍: 0 ⏩: 1
The math is based on Moebius transformations in complex plane.
They are genuine 3D hyperbolic transformation in 3D hyperbolic space but
observed on the boundary of the Poincare ball (analog of Poincare disk
for 3D hyperbolic space). The boundary of Poincare ball is projected
onto plane via stereographic projection.
So all the fishes are identical as they are in original Poincare disc view
in M.C.Escher woodcut.
👍: 0 ⏩: 0
Looks like it has 180 degree rotational symmetry?
I had thought it was circles intersecting the rectangle boundary at 90 degrees, somewhat like a poincare disk. But the big "circles" in the lower right and upper left look decidedly elliptical.
👍: 0 ⏩: 1
All circles were originally circles, but after conformal mapping (Mercator projection) they were a little squashed.
👍: 0 ⏩: 0