HOME | DD
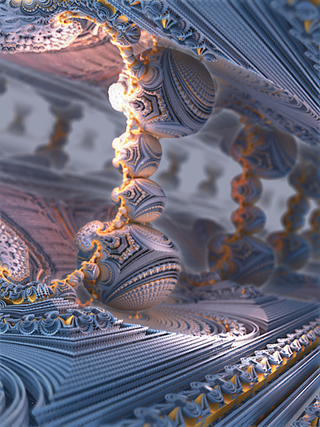
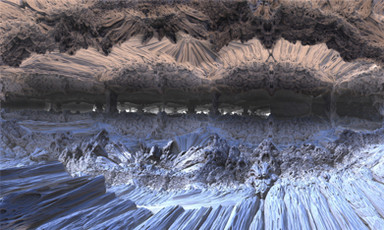
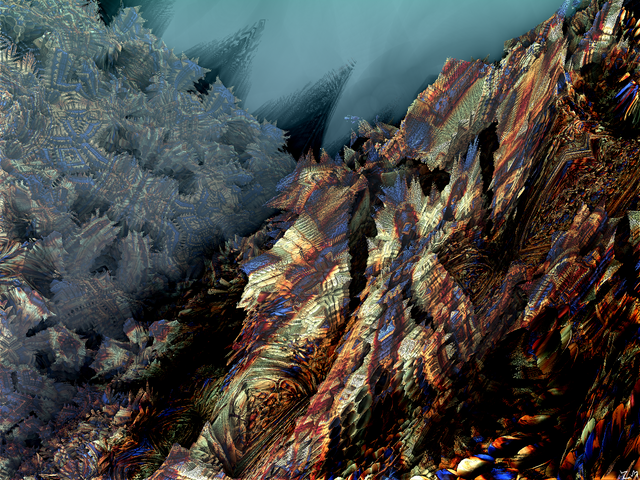
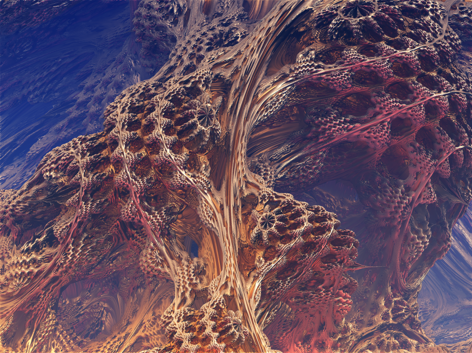
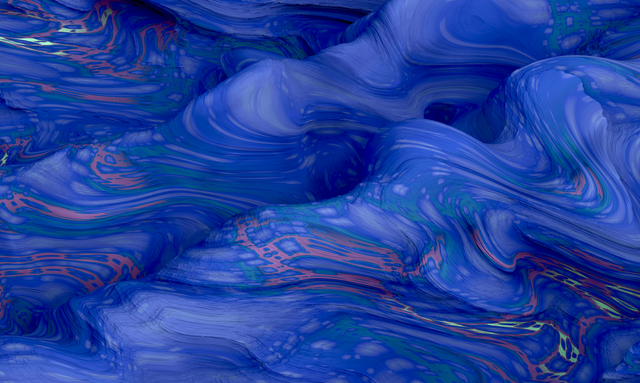
 MakinMagic — Bridges
MakinMagic — Bridges
Published: 2009-10-07 16:44:04 +0000 UTC; Views: 7978; Favourites: 16; Downloads: 110
Redirect to original
Description
Created using Ultrafractal.Related content
Comments: 17

I have theoretical knowledges of iteration process P-->P^[8] + C
but inner points, holow and solid, are a mistery for me
Please, how all the inner points (holow and solid) into Mandelbulb are defined?
👍: 0 ⏩: 1

As the Mandelbulb formula is iterated the value P in the form you gave will either tend to infinity or not. The empty space around the Mandelbulb consists of all the points in space where the value tends to infinity. the solid surface if done perfectly would consist of all the boundary points between those that tend to infinity under iteration and those that do not do so. In practice however getting the exact boundary is not possible as it would require infinite iterations. There are usually 2 ways of deciding when this surface (boundary) is reached - the older method is simply based on reaching a given iteration count before the value (P) gets beyond a certain limit (representing infinity). This limit can generaly be 2 or higher but in practice the larger the value the better but obviously the slower the calculation since the more iterations are required before the test value is reached. In 3D rendering a ray-marching technique is used on rays from the viewpoint until a point on the ray is found to be solid i.e. it's iterations reached maximum before the test bailout (limit) was reached - all the empty space are points where the test bailout was reached before reaching the maximum iteration count. This method either has to use brute-force stepping or very approximate stepping along the rays cast to accurately find the surface - nowadays most surfaces are foud using ray marching using the distannce estimation calculation - this is too complex to detail here but basically extra calculations during iteration can give you an approximate minimum distance to solid from any given point in the fractal space, allowing you to step that distance (or a fraction thereof allowing for approximtion errors) along the rays knowing that you haven't yet reached the surface. If the distance estimation for a given point is ess than a given threshold then it's assumed to be accur5ate enough to be considered as asurface point - obviously the higher magnification or higher resolution required then the smaller this theshold needs to be and there are limits even when using double, even more so if using just float (as in fragment shader code).
👍: 0 ⏩: 0

It gives the impression we can lose ourselves in the landscape. I like it a lot.
👍: 0 ⏩: 1

You're very much welcome.
👍: 0 ⏩: 0

reminds me of those ice bridges from the ice age waaaaay back in the day, you know the ones that supposedly linked north america with eurasia across the bering strait?
👍: 0 ⏩: 1

yeah you know your history too eh my friend?
👍: 0 ⏩: 0

You remind me of ~RCPage . He explored Apo and now Incendia, and pushed as far as he could.
👍: 0 ⏩: 1

We're all only just started with respect to investigating this one
👍: 0 ⏩: 1