HOME | DD
 Reflective-Sentinal — VIDEO! 2+3 point perspective find squares cube
Reflective-Sentinal — VIDEO! 2+3 point perspective find squares cube
Published: 2012-06-01 05:17:09 +0000 UTC; Views: 2787; Favourites: 27; Downloads: 153
Redirect to original
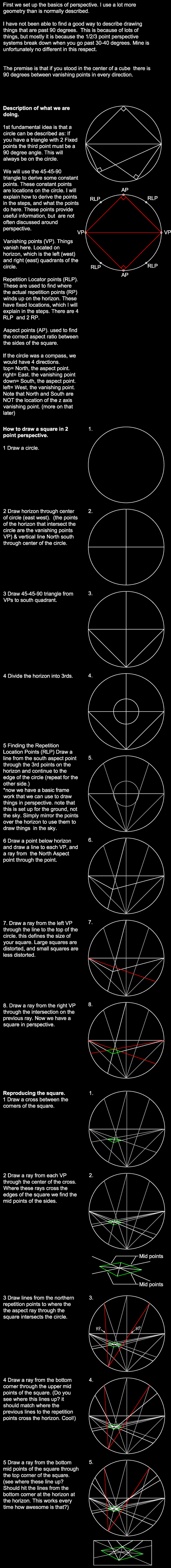
Description
*Edit* I have made a video [link] that explains how to use these concepts more clearly than this tutorial does.Monguro [link] suggested a book to be [link] that had a method of describing 3 point perspective that is remarkably similar to what I have here. The methods are very well presented in that book, and in practice are probably great for actually drawing, but are geometrically not sound.
I have been able to modify my 2 point solution to work for the 3 point solution.
Still don't have a great explanation for where the 3rd point goes, but I have a narrower range than I had before. (The 3rd vanishing point is constrained in the left right dimension by the first two vanishing points, it is almost unconstrained in the up down dimension. The only exception is the area near the horizon, it cannot be within the circle drawn between the two vanishing points.) As much as the idea bothers me, the start of a drawing is a line for a horizon, and then a 3rd vanishing point (if you are close to the object, the point should be close to the horizon. If you are far from the object, the vanishing point is far from the horizon. This has to do with how things distort when they occupy a wide angle of your vision. I do not have a solution worked out for this so just use your judgement.
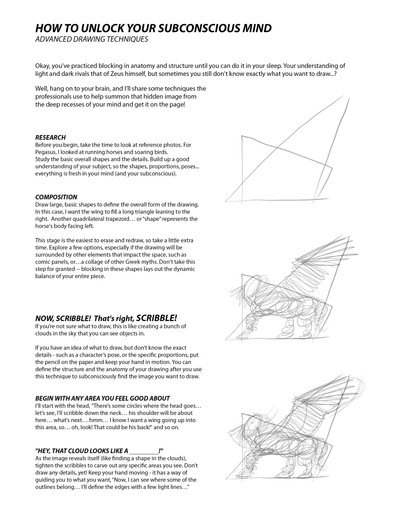
[Drawing 3 point perspective.:]
1) Draw a circle. Draw a line through the centre of the circle, where the line intersects the circle defines out 2 vanishing points. Locate a 3rd vanishing point above or below this horizon, outside of the circle and horizontally constrained between the first two points.
With the two vanishing points on the horizon, and 3rd vanishing point, we have a triangle.
2) We will now treat each side of this triangle as a horizon, and draw the 2 point square solution up till step 5.
3) Draw the true vertical:
Draw a line perpendicular to the horizon from the 3rd vanishing point to the horizon. This is our true vertical. Due to a quirk of geometry (the parallel objects converge rule), this will always be in our centre of vision, and for the purposes of illustration, in our picture.
you can test this by looking at a tall blocky building. When you look at the ground level, the building is wider that it appears at the top, and when you look at a specific corner, it is exactly vertical. But when you focus on one vertical, pay attention to how the far side of the building gets closer to the corner that you are focusing on, it looks a little tilted doesn't it?
4) Start drawing the object:
By locate a point on the true vertical, and drawing rays through it from the 3 aspect points to the their respective circles.
5) Locate the (6) repetition points.
Draw lines from where the aspect lines intersect with their circles back to their repetition locator points. Where these lines cross the triangle is where the Repetition points are.
6) Draw your cube.
Draw a line from each vanishing point to our object point. Measure off how big you want your cube on one of the these lines, and draw a line from that length back to the appropriate vanishing points. draw lines from vanishing points through the intersections of the aspect lines, to find squares. repeat for all three sides of the front of the cube. Continue for the back if necessary.
And dang-it. noticed something else. What I thought were aspect points are actually aspect locator points. the aspect points are where the line crosses the triangle. At least it is consistent





Related content
Comments: 33

I try it more than a couple times and failed at stage 4 with dividing into 3rds the other thing is the position of RLP within the circle
The video on youtube is better but also kind of confusing ...or just to fast at the end at 2:50
👍: 0 ⏩: 2

I have a hard time simplifying things. I always want to explain everything at the same time. Most of the stuff I have already put up sucks because of this.
I have tried again to explain the perspective of a cube, and have it simplified to 3 main steps.
[link]
👍: 0 ⏩: 0

Hmm. Can you show me what you have? I will go back and try to make another stab at a tutorial some time soon once I understand what I screwed up.
Because I am posting stuff as I develop an understanding of it, the result is a bit fragmented and confused unfortunately. Yea, the end is very fast. It does however just go back over the same stuff as the beginning.
👍: 0 ⏩: 1

Sure, in general it's the position of RLP within the circle, just cant figure it out.
thats what I got at the moment
[link]
👍: 0 ⏩: 0

While this is a great source to study for perspective, Ive found the deeper you look into it the more I understand less.
[link]
this is a great book on perspective. As an artist, the simpler it is the easier my job becomes in trying to understand it
👍: 0 ⏩: 1

I find that things get simpler when I understand them. It is not that I do not understand how perspective works, it is that there are questions that the typical perspective does not answer.
Here are some of these questions:
How to find a square in 2 point perspective? (solved for the moment. It is quite probable that I decide I was wrong and a different solution is better.)
Where is the 3rd vanishing point placed? (There is more than 1 3rd vanishing point [link] . Which may explain why understanding things related to the 3rd vanishing point is so hard.)
How to find a cube in 3 point perspective? This question is directly related to where the 3rd vanishing point is. Answering this question will let us measure things in the 3 axes.
I am curious to read about solutions to these questions, but I am not eager to buy books if they do not advance my understanding of the geometry of perspective. Does this book answer these questions?
👍: 0 ⏩: 1

I wont knock off you trying to understand the complexity of perspective but imo when taken to mathematical heights it becomes mechanical as opposed to natural. Theres a section of the book that does a good job of illustrating mechanical perspective without sounding like a math textbook in some cases, which is what as an artist, is more useful.
also to answer your 3rd perspective question, personally i think it goes wherever you want it to go to fit your composition. Its not a natural occurring perspective as I only know of it to occur when looking at things from an extreme low or high angle.
👍: 0 ⏩: 1

As I understand perceptive, 3 point perspective is the general case and 1 and 2 point perspective are special exceptions created by perpendicularity from 1 or 2 axes.
I think there may a specific place the third point should go, But I don't know how to find it yet. Like I said, there are multiple vanishing points in theory, if I did the geometry right.
I wouldn't dream of using all of this with a pencil to set up a drawing, it is just far to large. But I will have a better idea of where the points should be going, and that will make a more convincing picture.
Thanks for the discussion.
👍: 0 ⏩: 0

I'm very impressed by the lengths that you are going to, to really understand perspective. The two books that offer explanations that might be more satisfying to you, that I have found are:
[link]
and the follow up book
[link]
They go into depth about the how and the why and offer diagrams you can follow to understand some of the math as well as practical applications.
For me perspective always feels like dark wizardry and though I long to draw beautiful buildings, I know that basically learning how to draw cubes and circles in perspective is the basic building block that I haven't 100% mastered.
👍: 0 ⏩: 1

Thank you.
I just want to know the theory of how it really works before I start taking short cuts.
I always appreciate hearing about books that I can read on the subject. I will see I can find these someplace local that I have a look at them before I buy them.
From the Amazon preview, I cannot tell whether it would cover what I am looking to learn. It would cover techniques for how to draw the stuff, but I do not know if it would cover how to find squares on the vertical sides of objects / how to decide where the 3rd vanishing point goes.
Don't worry about perspective too much. If you have any big stone churches or buildings in your area, spend some time sketching them. The repetition of shapes and large open spaces are always hard to find in other architecture. Other than that, sketch street corners, its a great place to get 2 point perspective.
👍: 0 ⏩: 1

These books are very thorough but I do think its a good idea to see whats in them before you buy. If you can't find them in your area, I'll try to get a hold of the copier scanner at work and make a copy of a chapter for you from each.
👍: 0 ⏩: 1

I have not been able to find it locally. The chapter on 3 point perspective from the first book will let me judge if it is able to teach me what I need to know.
👍: 0 ⏩: 1

I'll send you a note with a link to the chapter.
👍: 0 ⏩: 3

hmm. Now that I have slept on it, I realize that the centre of vision will never be outside of the circle.
So it does provide useful cubes. YAY!
This solution is independent of the location of the 3rd vanishing point, and does not provide a way of locating the 3rd vanishing point.
I can just do some testing to find a few 3rd vanishing point cases to establish a general guideline for close med far.
👍: 0 ⏩: 1

Glad that you're getting something out of it.
👍: 0 ⏩: 1

I ordered the book today.
His method of determining the centre of view is incorrect. Very close, but incorrect because it is not repeatable on all sides of the triangle. His idea of gravity lines is interesting but not that useful geometrically. His proof is true for an equilateral triangle, and the farther you deviate from that, the more it breaks down, because the lines that control the aspect ratio of the squares are not perpendicular to the 3 horizons. This is where my solution to finding a square in 2 point perspective becomes useful.
I need to do a bit more to prove a more correct solution, But I thinks I has it. *My preecioussss*
Damn solution is taking a billion lines to work through though.
👍: 0 ⏩: 1

I can imagine lol!! Looking forward to your finished solution.
👍: 0 ⏩: 1

I am actively updating the description, but... It is hard to articulate. I have realized how elegant the solution I found is, but explaining it is hard.
The principals are simple though.
👍: 0 ⏩: 0

hmm. I like and dislike things about how the info is presented. He certainly knows his stuff though. I see a lot of my solution above reproduced in this document. (Aspect lines = gravity lines)
I am going to be running these ideas through autocad to see if they work. I have tested similar ideas and found problems. It does not have a solution or guideline for the placement of the third vanishing point.
It suggests a solution for measuring the axes of the cube and I was able to use this to interpret a range of possible values for the 3rd vanishing.
/////////////////////////// testing:
The system breaks when the centre of vision is outside of the circle defined by the two vanishing points on the horizon. I will try to get a description of the distance to make this happen.
👍: 0 ⏩: 0

Cool, I will look forward to it.
👍: 0 ⏩: 0

Looks great, i gonna read/study this tomorrow. I rly need to learn more about perspective
👍: 0 ⏩: 3

Fixed it. It should make more sense now.
👍: 0 ⏩: 1

Fixed it more. now it has 3 point perspective solution.
👍: 0 ⏩: 0

and a large portion of what I had figured out is wrong. Damn.
👍: 0 ⏩: 0

BE WARNED!
I am taking a non standard approach to perspective because the usual explanations don't make a lot of sense to me.
Some of the stuff I have noticed here is stuff I have never seen in any of the tutorials or books I have bought.
👍: 0 ⏩: 0

I've done an exercise in three-point perspective as well: [link]
👍: 0 ⏩: 1

Interesting. Do you understand why the vanishing points get placed where they are?
👍: 0 ⏩: 1

Well, in my case, I simply placed the vanishing points in an equilateral triangle. Are you talking about your picture?
👍: 0 ⏩: 1

Mean vanishing points in general. How do you decide the specific positions for the 3 point?
👍: 0 ⏩: 1

That's the thing. I think that when you're dealing with multi-point perspective, the positions are arbitrary.
👍: 0 ⏩: 1

That is where I became dissatisfied with the traditional description of how perspective works. As far as perspective goes in describing cubes etc. It is not art, it is geometry and math. There is usually only a single right answer in math, but there can be many ways to get there.
If you trace the viewpoints from a picture of a building, standing at a particular spot, the vanishing points will always be in the same place. (assuming same lens etc)
Thank you for discussing this with me, I appreciate it.
👍: 0 ⏩: 1

Usually multi-point perspective disorients me because the human eye is used to seeing in one-point (or linear) perspective.
👍: 0 ⏩: 1

We have a large part of our brain dedicated to the processing information from our eyes, distinguishing the parallax of the images derive distance. It is how we are able to catch balls and flying objects without being able to compare them to other objects in.
Single point perspective seems to me to be the rarest form of perspective. When I look at my desk I see it in 3 point perspective because it has 3 major dimensions with parallel lines.
1 point perspective is 3 point perspective where 2 of the axes (apparently that is the plural of axis) of the object are perpendicular to the viewer. these perpendicular axes still have vanishing points, but because the axes are perpendicular to the viewer our eyes have trouble distinguishing the perspective, which leads us to representing it as a straight line.
If you look at the classic 1 point perspective drawing of a road vanishing into the horizon, what can you see if you look straight down at the road? are the edges parallel? are they straight? This is where perspective seems to merge with calculus, as I understand it. When you think about it, you are not seeing a straight line, you are seeing a curved line that your brain interprets as straight. when you look down in this example, the visually widest part of the road is the piece that is at your feet, and every foot farther away from you is smaller, and thus closer to to the horizon.
👍: 0 ⏩: 0