HOME | DD
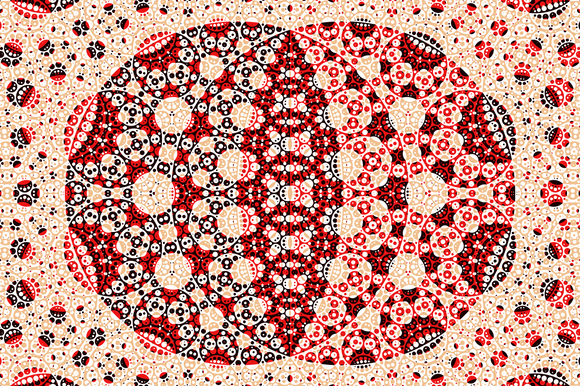
Vladimir-Bulatov — Self similar 'Circle limit I'
by-nc-sa
Published: 2012-02-23 07:20:47 +0000 UTC; Views: 3698; Favourites: 30; Downloads: 197
Redirect to original
Description
M.C.Escher "Circle Limit I" getting smaller and smaller..Related content
Comments: 11
You're very welcome!
More! More! More!
👍: 0 ⏩: 0
Omg, crazy
I really would like to see a piece of your code.
I write formulas too, and I would love to implement some of your concepts, especially the "normal" circlelimit. I only found partial implementations on the internet. I assume you use a single iterative formula (not a big memory stack) else I can't use it
Luca
👍: 0 ⏩: 1
I think few people ([link] ) implemented some hyperbolic tilings in a Apophysis
and Mandelbul3D.
I not quite know yet how these programs work to be able to provide
formulas. It all are Moebius transformations.
👍: 0 ⏩: 1
I did an apollonian fractal, it is the same? Or needs a log()? 
👍: 0 ⏩: 1
Apollonian gasket is made by reflections in 3 circles, which touch each other.
Hyperbolic triangle tilings are made by reflections in 3 circles with intersection angles pi/k, pi/m, pi/n.
This image is generated by reflections in 5 circles.
4 circles make a chain with intersection angles pi/2,pi/2, pi/2 pi/3. The angles of intersection of 5th circle with other four are pi/2,pi/2,pi/2,pi/4.
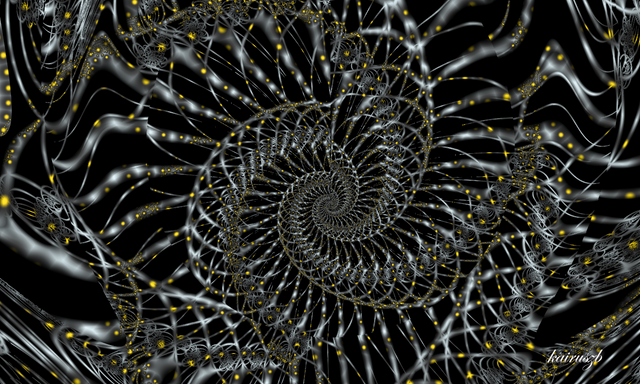
In addition a conformal mapping (cirlce to a band) is applied to stretch two selected limit points to +-oo and exponential mapping is applied to wrap image into a spiral.
The idea of bended quadrilateral hyperbolic kaleidoscope is described at my site http:/bulatov.org/math/1107
The fifth circle (hyperbolic plane) adds nice fractal twist.
👍: 0 ⏩: 1
Striking thanks! I am not understanding much but thanks
👍: 0 ⏩: 0